The Conic Sections
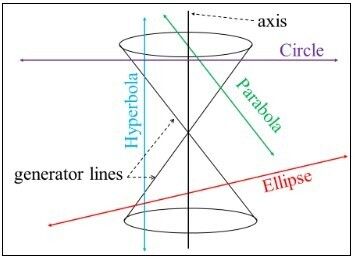
Simply put, a Conic Section is the intersection of a plane and a right-circular cone (two single cones placed apex-to-apex) as shown in the diagram at the left. By changing the angle and location of the intersection, one can produce a circle, an ellipse, a parabola, or a hyperbola. If the plane is perpendicular to the axis of the cone, a circle is produced (see purple line in the diagram). If the plane is parallel to one of the cone’s generator lines, a parabola is produced (see green). For a plane not perpendicular to the cone’s axis, not parallel to the cone’s generator lines, and intersecting only the lower (or upper) cone, an ellipse is produced (see red). And finally, for a plane intersecting both upper and lower cones, a hyperbola is produced (blue).
The animations below are presented in pairs. The first of the pair is the basic geometric construction that either generates the Conic or demonstrates one of its properties. The second of the pair is my “artistic rendering” based on the first of the pair.
The animations below are presented in pairs. The first of the pair is the basic geometric construction that either generates the Conic or demonstrates one of its properties. The second of the pair is my “artistic rendering” based on the first of the pair.