Lemniscate of Bernoulli
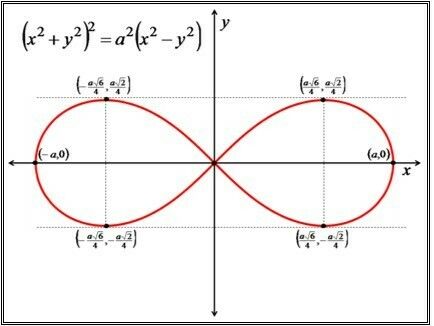
The curve called the Lemniscate of Bernoulli is depicted to the left along with its equation. More details about this curve can be found in my book Playing with Dynamic Geometry, Chapter 15.
This curve is named after Jakob Bernoulli, an eminent 17th century mathematician and member of a famous family prominent in the sciences. He described the curve as being shaped like a figure eight, a knot, or the bow of a ribbon. Following the protocol of his day, he gave this curve the Latin name of lemniscus, which translates as a pendant ribbon to be fastened to a victor's garland. Today, we know the curve as the Lemniscate of Bernoulli (pronounced lem·nis·cate).
The animations below are presented in pairs. The first of the pairs is the basic geometric construction that either generates the Lemniscate or demonstrates one of its properties. The second of the pairs is my “artistic” rendering based on that basic construction.
This curve is named after Jakob Bernoulli, an eminent 17th century mathematician and member of a famous family prominent in the sciences. He described the curve as being shaped like a figure eight, a knot, or the bow of a ribbon. Following the protocol of his day, he gave this curve the Latin name of lemniscus, which translates as a pendant ribbon to be fastened to a victor's garland. Today, we know the curve as the Lemniscate of Bernoulli (pronounced lem·nis·cate).
The animations below are presented in pairs. The first of the pairs is the basic geometric construction that either generates the Lemniscate or demonstrates one of its properties. The second of the pairs is my “artistic” rendering based on that basic construction.