CISSOID OF DIOCLES
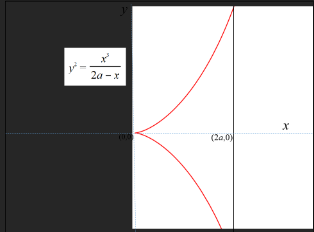
Supposedly, in 430 BC, the Athenians were suffering from a terrible plague. In order to appease the gods and stop the plague, the oracle of the god Apollo at Delos was consulted. The Athenians were instructed to double the size of their altar, which at the time, was a cube. Armed only with a straightedge and compass, they proceeded to double every edge, but, of course, this increased the volume of the cube by a factor of eight and the ravages of the plague increased. After some thought, they came to the observation that the problem consists of constructing a length that is the cube root of 2 times the length of an edge of the original altar. As a result, the problem of duplicating the cube using only compass and straightedge became known as the Delian problem. As it turns out, one can never find a solution requiring only the straightedge and compass, as was proven some centuries later. However, the Greeks obtained many solutions using a variety of clever techniques. Special curves were invented and investigated for this purpose, which themselves could not be constructed by using only a straightedge and compass. One such curve investigated by Diocles (circa 180 BC) in connection with this Delian problem is a curve that is today called the Cissoid of Diocles (a graph of which is shown on the left); cissoid means ivy-shaped.
The animations presented below are in pairs. The first of the pair is a basic construction that either generates the Cissoid or demonstrates a property of the Cissoid. The second of the pair is an attempt to create an interesting dynamic animation with all of the underlying mathematical construction hidden.
The animations presented below are in pairs. The first of the pair is a basic construction that either generates the Cissoid or demonstrates a property of the Cissoid. The second of the pair is an attempt to create an interesting dynamic animation with all of the underlying mathematical construction hidden.